Economical cycles in dynamics

Technology

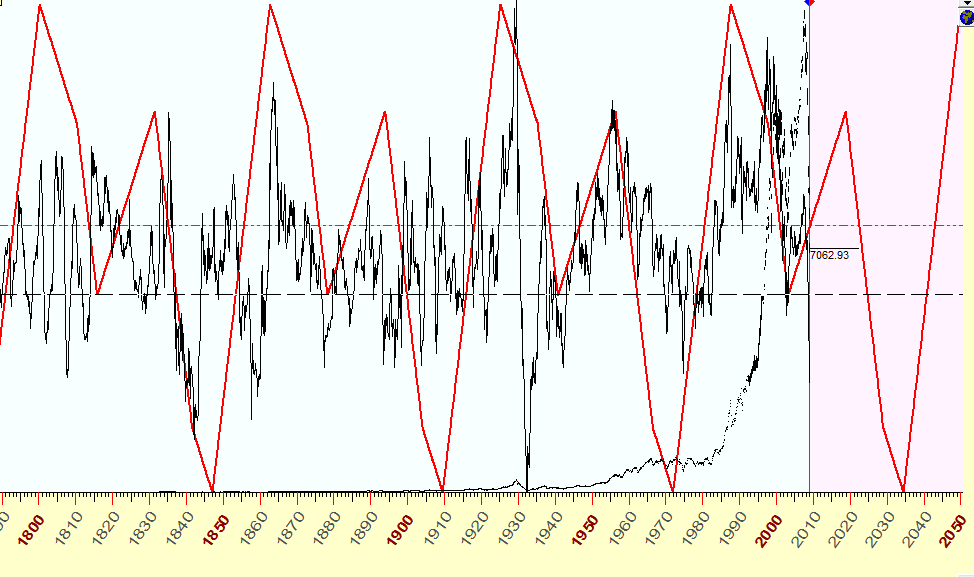
Here I do a sample of the cyclic analysis for the longest financial data set that I have: DJI from the year 1789 year till now. We can take this file as the history of the American stock market. To reveal the cycles, the advanced mathematical approach is used - the wavelet analysis.

Why do we do the wavelet analysis?

The answer is simple: the wavelet analysis allows to analyze the life of cycles in dynamics, and this fact is extremely important in dealing with the financial data. For example, we can see here the presence of one of the strongest economical cycles, 9-11 years Juglar cycle. It is present practically in all types of financial data, for almost all financial instruments. It is quite possible that it correlates with 10-year Decennial cycle. This cycle is my favorite cycle. It has worked very well for more than a hundred years, since the end of 19th century till the end of 20th century.

It was so before, but not now. It looks like the energy of this cycle is weakening, and its energy is divided between two cycles with the periods of 8.9 years and 9.9 years (see the diagram below).

Now let's do it, let's see the dynamics of these cycles. 

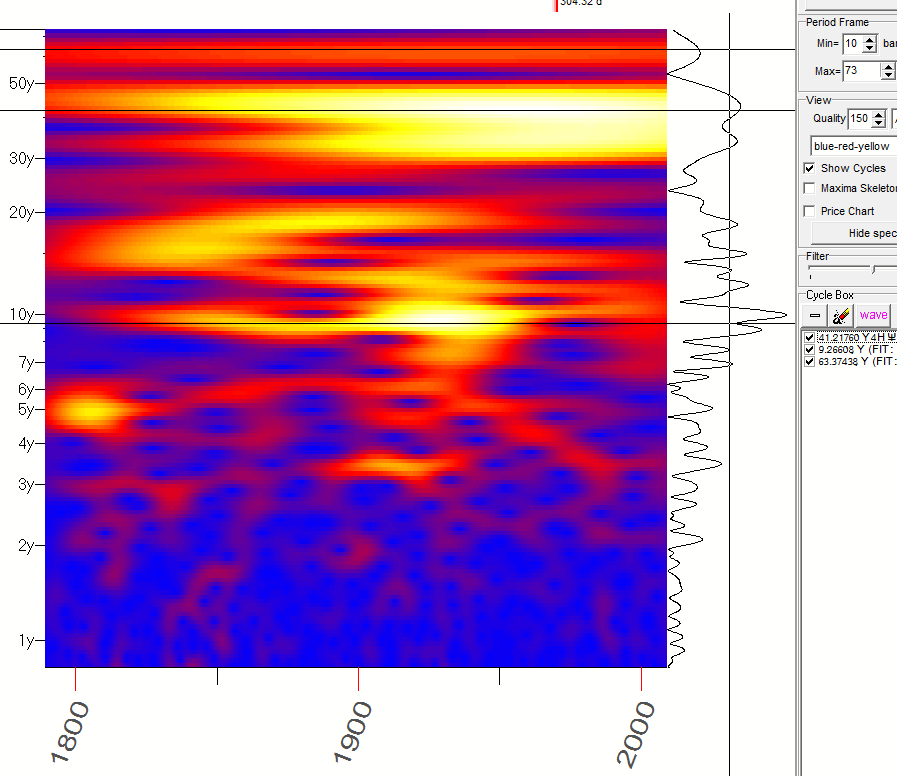
This is the wavelet diagram calculated for DJI since the year 1789 till 2010:

The hot red-yellow zones represent the zones with some active cycles. Axis X represents the time (the length of the cycle\s activity) while Y axis represents the period of the analyzed cycle.

What cycles do we see here? I would like to discuss some of them.

10 years Juglar cycle

Particularly  this horizontal bright red stripe represents 9-11 years Juglar cycle:

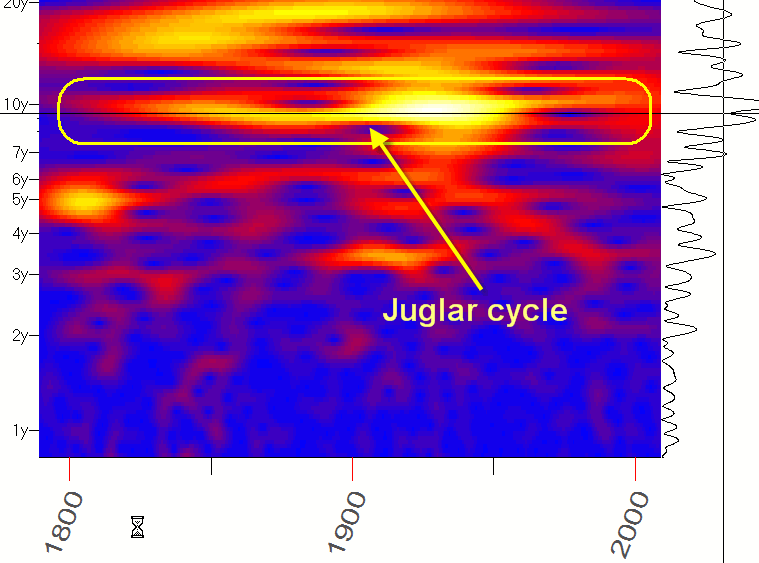
As you can see, the brightest part of this diagram falls to the years 1900-1950, i.e.  the economic/stock market gears of this period have been ruled by this cycle.

And starting from 1960-1970 this cycle is weakening. Now it is divided on two cycles:

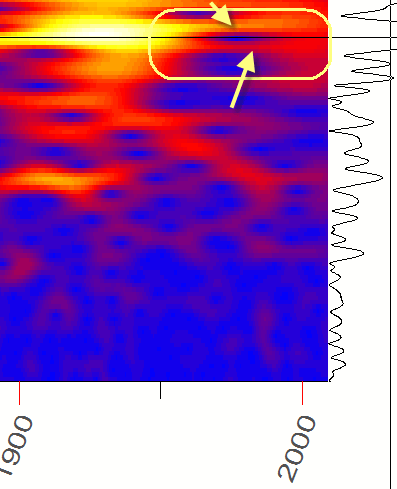
The division is associated with the year 1973. It was the first year of a new economic life without the gold standard and with floating currencies instead, as well as constantly increasing oil demand and oil prices.

41 years oil cycle

Now let's look at the brightest stripe there. It represents 41-year cycle:

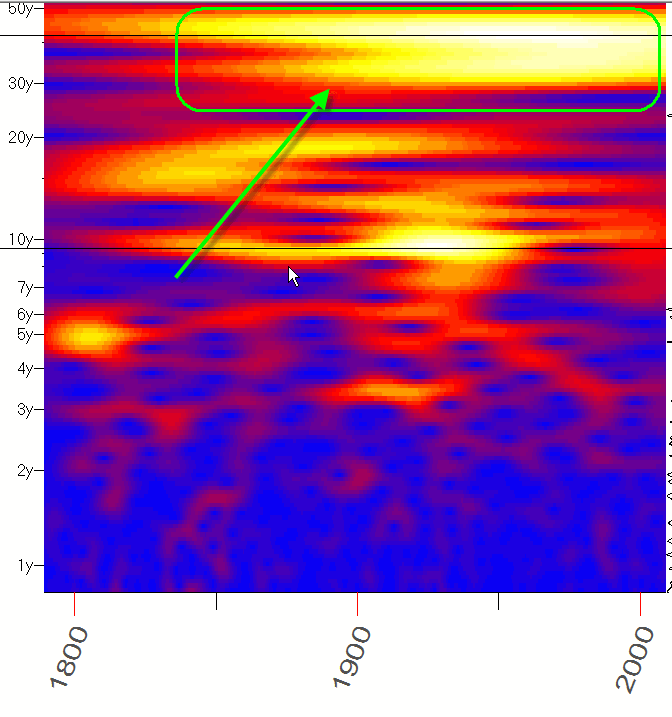
As you see, this stipe is very bright since the end of 19th century - the beginning of 20th century. Besides, it has started as two cycles, with the periods of 33 and 43 years; then the energy of these two cycles was combined into one 41-year cycle.

It means that, staring from 1900s, some new economical gears have been involved; they make one cycle with the period of 41 year.

What does this cycle mean?

I don't know. I can only guess, because more price history has to be analyzed to get the whole picture.

The first hint I see is the period of this cycle. It is 41 years. This is a quarter of a complete Neptune  cycle. Astrologically Neptune is connected with the oil. So it is quite possible that this 41-year cycle represents the oil era of the world economy; at least, everybody knows that the oil plays an extremely important role in the economy of the 20th century.

Pay attention to the fact that 41-year cycle is weakening as well:

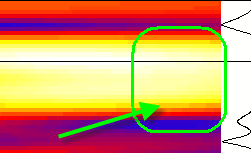
This weakening becomes obvious within the last 10 years (2000-2010). And if we do the extrapolation of this shape, this cycle should lose its energy  in the years 2020-2025.

Consider this information as well: according to one research, the massive switch to hybrid/electric cars should happen around the year 2020 year. Maybe this will be the time of completion of this cycle and the time of birth of some new cycle that will represent new (though not known yet) economical gears.

In the past, this 41-year cycle has worked this way:

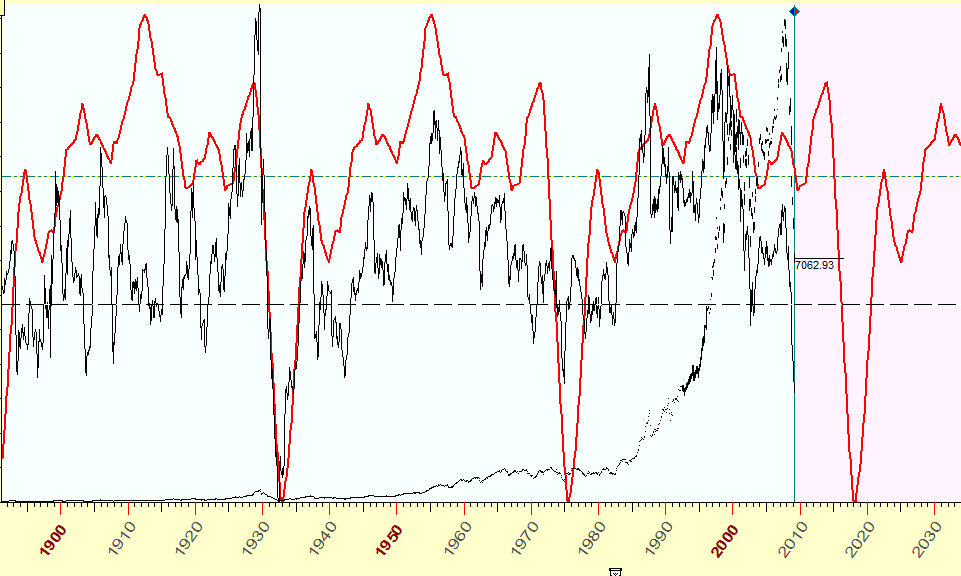
According to this cycle, a big drop is expected in the year 2018; however, I would be very cautious with such a forecast as this cycle may be dissolved before that time.

62-year Kondratiev cycle

This cycle is very stable; its intensity practically does not change within two hundreds years. On the wavelet diagram, this cycle is presented by this horizontal stripe:

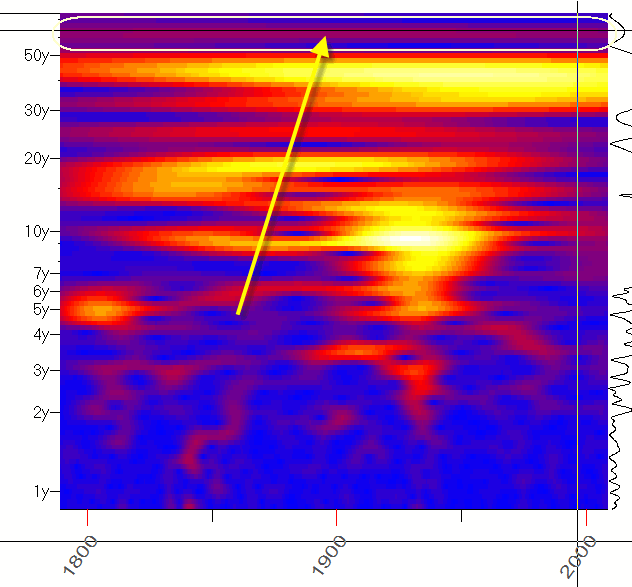
This cycle is weak, though it is very stable. This is how it works in time: